Chapter 5: An Overview of RF Filter Design
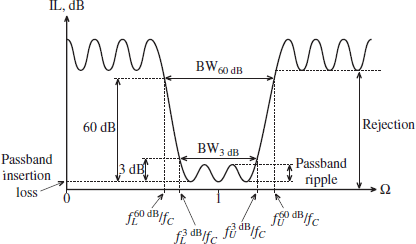
Capitalizing on our knowledge of networks developed in Chapter 4, we are ready to extend and apply these concepts to design RF filters. It is of particular interest in any analog circuit design to manipulate high-frequency signals in such a way as to enhance or attenuate certain frequency ranges or bands. This chapter examines the filtering of analog signals. As we know from elementary circuit courses, there are four types of filters: low-pass, high-pass, bandpass, and bandstop. The low-pass filter allows low-frequency signals to be transmitted from the input to the output port with little attenuation. However, as the frequency exceeds a certain cutoff point, the attenuation increases significantly with the result of delivering an amplitude-reduced signal to the output port. The opposite behavior is true for a high-pass filter, where the low-frequency signal components are highly attenuated, or reduced in amplitude, while beyond a cutoff frequency point the signal passes the filter with little attenuation. Bandpass and bandstop filters restrict the passband between specific lower and upper frequency points where the attenuation is either low (bandpass) or high (bandstop) compared to the remaining frequency band.
In this chapter, we first review several fundamental concepts and definitions pertaining to filters and resonators. Specifically, the key concept of loaded and unloaded quality factors will be examined in some detail. Then, we introduce the basic, multisection low-pass filter configuration for which tabulated coefficients have been developed both for the so-called maximally flat binomial, or Butterworth filter, and the equiripple, or Chebyshev filter. The intent of Chapter 5 is not to introduce the reader to the entire filter theory, particularly how to derive these coefficients, but rather how to utilize the information to design specific filter types. We will see that the normalized low-pass filter serves as the basic building block from which all four filter types can be derived.
Once we know the procedures of converting a standard low-pass filter design in Butterworth or Chebyshev configuration into a particular filter type that meets our requirements, we then need to investigate ways of implementing the filter through distributed elements. This step is critical, since at frequencies above approximately 500 MHz lumped elements, such as inductors and capacitors, are unsuitable. Relying on the Richards transformation, which converts lumped into distributed elements, and Kuroda's identities, we are given powerful tools to develop a wide range of practically realizable filter configurations.
